C/1942 C1 Whipple-Bernasconi-Kulin
more info
Comet C/1942 C1 was discovered independently by three observers: by Fred L. Whipple on 25 January 1942 (Harvard Observatory, Massachusetts, USA), by Giovanni Bernasconi (Como, Italy) on 10 February, and by György Kulin (Konkoly Observatory, Budapest, Hungary) on 13 February. Soon, prediscovery images (exposed on 28 December 1941 and 17 January 1942) was found by Whipple. At the moment of discovery, this comet was about three months before perihelion passage, and it was last seen on 8 January 1943. [Kronk, Cometography: Volume 4].
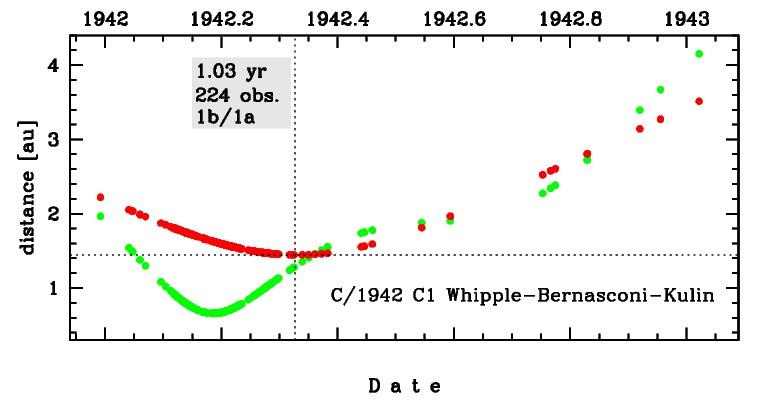
This comet made its closest approach to the Earth on 9 March 1942 (0.659 au), that is about a month after its discovery.
Solutions given here are based on data spanning over 1.03 yr in a range of heliocentric distances from 2.222 au through perihelion (1.445 au) to 3.513 au.
Pure gravitational orbit determined from the available positional measurements (224 observations) give 1a-class orbit.
It was possible to determine the non-gravitational orbit for C/1942 C1 (preferred orbit) where a noticeable decrease of RMS was obtained (from 1.46 arcsec to 1.23 arcsec).
This Oort spike comet suffers moderate planetary perturbations during its passage through the planetary system that lead to escape the comet from the solar systemon a hyperbolic orbit (see future barycentric orbits for both models of motion).
More details in Królikowska et al. 2014.
This comet made its closest approach to the Earth on 9 March 1942 (0.659 au), that is about a month after its discovery.
Solutions given here are based on data spanning over 1.03 yr in a range of heliocentric distances from 2.222 au through perihelion (1.445 au) to 3.513 au.
Pure gravitational orbit determined from the available positional measurements (224 observations) give 1a-class orbit.
It was possible to determine the non-gravitational orbit for C/1942 C1 (preferred orbit) where a noticeable decrease of RMS was obtained (from 1.46 arcsec to 1.23 arcsec).
This Oort spike comet suffers moderate planetary perturbations during its passage through the planetary system that lead to escape the comet from the solar systemon a hyperbolic orbit (see future barycentric orbits for both models of motion).
More details in Królikowska et al. 2014.
| solution description | ||
|---|---|---|
| number of observations | 224 | |
| data interval | 1941 12 28 – 1943 01 08 | |
| data type | perihelion within the observation arc (FULL) | |
| data arc selection | entire data set (STD) | |
| range of heliocentric distances | 2.22 au – 1.45 au (perihelion) – 3.51 au | |
| type of model of motion | NS - non-gravitational orbits for standard g(r) | |
| data weighting | YES | |
| number of residuals | 411 | |
| RMS [arcseconds] | 1.23 | |
| orbit quality class | 1b | |
| next orbit statistics, both Galactic and stellar perturbations were taken into account | ||
|---|---|---|
| no. of returning VCs in the swarm | 0 | |
| no. of escaping VCs in the swarm | 5001 | |
| no. of hyperbolas among escaping VCs in the swarm | 5001 | * |
| next reciprocal semi-major axis [10-6 au-1] | -786.22 – -774.92 – -764.38 | |
| next perihelion distance [au] | 3.005 – 3.029 – 3.054 | |
| synchronous stop epoch [Myr] | 0.684 | S |
| percentage of VCs with qnext < 10 | 100 | |
Time distribution of positional observations with corresponding heliocentric (red curve) and geocentric (green curve) distance at which they were taken. The horizontal dotted line shows the perihelion distance for a given comet whereas vertical dotted line — the moment of perihelion passage.
| next orbit statistics, here only the Galactic tide has been included | ||
|---|---|---|
| no. of returning VCs in the swarm | 0 | |
| no. of escaping VCs in the swarm | 5001 | |
| no. of hyperbolas among escaping VCs in the swarm | 5001 | * |
| next reciprocal semi-major axis [10-6 au-1] | -786.21 – -774.90 – -764.36 | |
| next perihelion distance [au] | 3.083 – 3.108 – 3.135 | |
| synchronous stop epoch [Myr] | 0.664 | S |
| percentage of VCs with qnext < 10 | 100 | |